Understanding Quantum Field Theory

Quantum field theory (QFT) serves as the foundation for the Standard Model of particle physics, which encompasses the theories of electroweak and strong nuclear interactions. Notably, quantum electrodynamics (QED) has achieved remarkable accuracy in predicting physical measurements. For instance, the magnetic dipole moment of the muon has an experimentally observed value of ( 233,184,600 (1680) times 10^{-11} ), while QED predicts it as ( 233,183,478 (308) times 10^{-11} ). This remarkable alignment is truly impressive.

However, QFT is also notorious for its divergences, particularly concerning vacuum energy density, often referred to as the vacuum expectation value (VEV). Each quantum field has a corresponding divergent zero-point energy, and summing over all modes—up to a plausible energy cutoff—results in an astronomically large vacuum energy density.

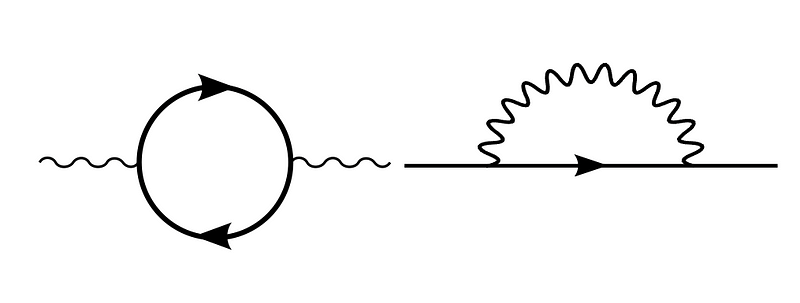
The disparity between the vacuum energy predicted by general relativity and the minuscule values observed experimentally is staggering, sometimes differing by as much as 120 orders of magnitude. This discrepancy is known as the cosmological constant problem, or vacuum catastrophe, and is highlighted by Hobson, Efstathiou, and Lasenby (HEL) as "the worst theoretical prediction in the history of physics."

The Vacuum Energy Dilemma

When gravity is factored in, this energy density creates significant issues. In the framework of general relativity, any form of matter or energy must be added to the vacuum energy, unlike other fields where it can be subtracted.

The first video titled "The Worst Prediction in Physics" explores the profound implications of these theoretical discrepancies.

A Brief Overview of Einstein’s Gravity Theory

Between 1915 and 1916, Einstein finalized his general theory of relativity, demonstrating that the curvature of spacetime results from the energy and momentum of surrounding matter and radiation.

The equation governing this relationship, expressed in terms of tensors ( G ), ( R ), and ( T ), reads:

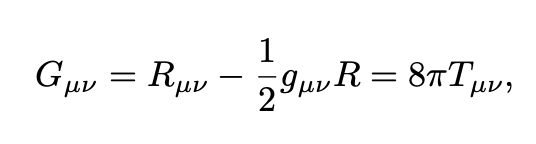
For those seeking a deeper understanding of these equations, refer to the article on:

Quantum Gravity, Timelessness, and Complex Numbers

The Ricci curvature tensor ( R ) measures the deviation of spacetime geometry from standard Euclidean space.

The tensor ( g ) represents the metric tensor and can be expressed as:

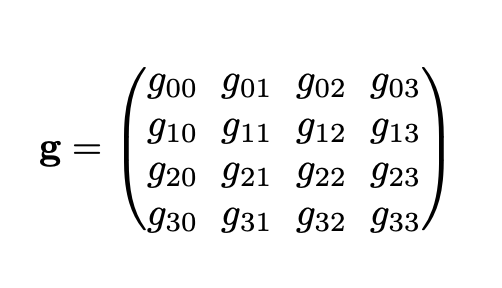
The corresponding line element can be denoted as follows:

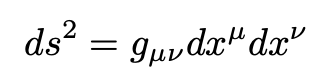
The tensor ( T ), located on the right side, is the energy-momentum tensor, which encapsulates the matter and energy characteristics that distort spacetime.

The Expanding Universe

In 1917, Einstein applied his general theory of relativity to the universe, then perceived merely as the Milky Way galaxy, in his groundbreaking work "Cosmological Considerations on the General Theory of Relativity." He envisioned a static universe with a closed spatial geometry.

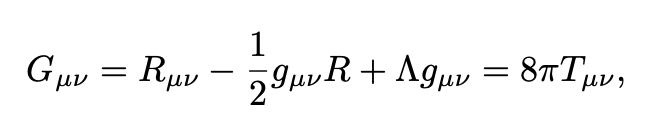
However, in 1929, Edwin Hubble uncovered that what were thought to be clouds of dust and gas were, in fact, distant galaxies, leading to the realization that the universe is expanding.

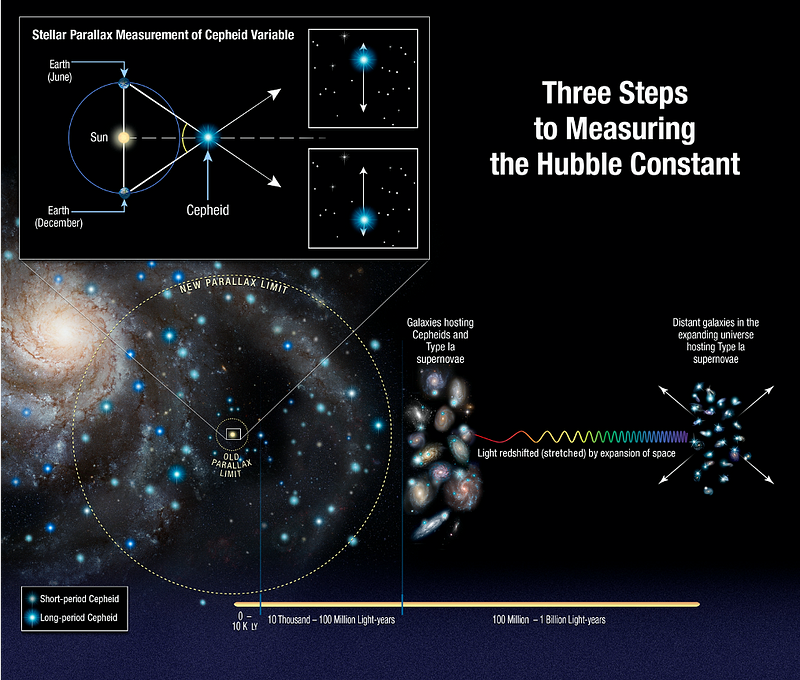
Hubble's findings, alongside contributions from Georges Lemaître, indicated that the universe's recessional velocity increases with distance, now known as Hubble's Law. This discovery rendered Einstein's cosmological constant unnecessary, prompting him to famously declare it "the biggest blunder" of his career.

The second video, "Worst Prediction in All of Physics - Vacuum Catastrophe," delves into the implications of these findings.

A New Perspective on the Cosmological Constant

Today, the cosmological constant is understood differently. It plays a crucial role in explaining the accelerated expansion of the universe, which is illustrated in the following figure.

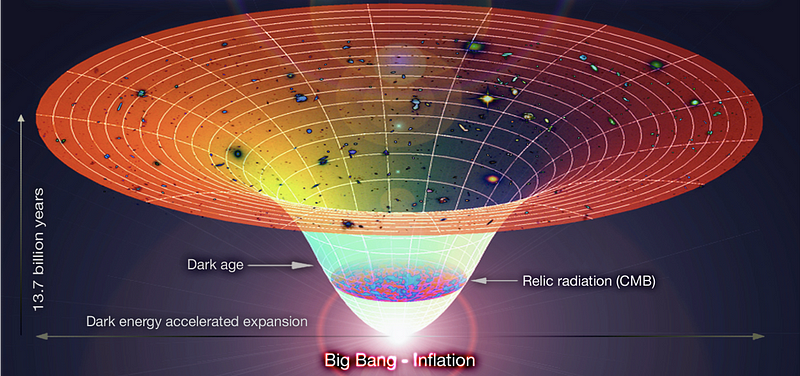
The Friedmann–Lemaître–Robertson–Walker (FLRW) Metric

When examining vast regions, like galaxy clusters, the universe's geometry appears approximately homogeneous and isotropic. This leads to solutions of Einstein's field equations known as FLRW universes.

As we explore these concepts, we arrive at equations that describe the dynamic interplay between matter, energy, and the expansion of the universe.

In summary, the challenges posed by the vacuum energy and cosmological constant remain among the most significant unsolved problems in modern physics, with ongoing research aimed at reconciling the theoretical predictions with observed phenomena.

Thank you for reading! Your thoughts and feedback are greatly appreciated. For more insights, feel free to check my personal website at www.marcotavora.me, as well as my profiles on LinkedIn and GitHub for additional content on physics and related fields!