Title: Unraveling the Mystery of Logarithms: An Engaging Algebra Challenge
Written on
Chapter 1: The Logarithmic Puzzle
I hope you appreciated yesterday's discussion about a fascinating mathematical clock. Today, we’ll delve into an intriguing algebra puzzle that revolves around logarithms.
Here’s a little hint: ?
I suggest you take a moment, grab your pen and paper, and try to solve it. Once you're ready, continue reading for the solution! ??
Solution
If you examined the hint, you might have noticed a telescope emoji. This leads us to the essence of the puzzle: a telescoping series!
A key property of logarithms is the change of base formula.

As demonstrated here, we can reformulate each term in our expression to reach the solution.
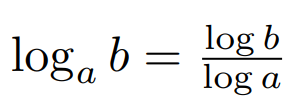
Do you see the direction we’re heading? In fact, all terms except for log(25), log(27), log(23), and log(3) will cancel out. This simplifies our expression to the following fraction:
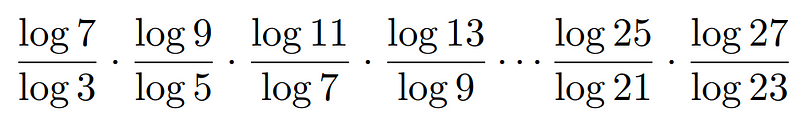
At this stage, our aim is to simplify the expression further. We can rewrite 25 as 5² and 27 as 3³.
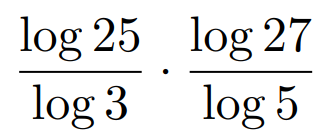
Thanks to the properties of logarithms, we can bring the exponents down in front.
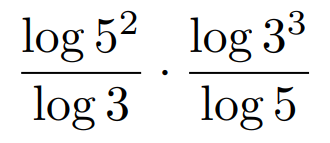
Now, all the logs neatly cancel, leaving us with 2 × 3, leading to our final answer:
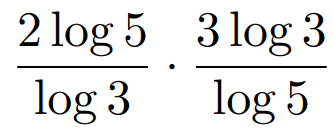
And there you have it!
Isn’t that amazing?
What was your thought process this time? I’d love to hear your insights in the comments below! 😊
This video, Logarithms - The Easy Way!, offers a straightforward explanation of logarithmic concepts, making them easier to grasp.
In this video, How to find the solutions when solving a logarithmic equation, you’ll discover techniques for solving logarithmic equations effectively.
Chapter 2: Engage with More Puzzles
If you enjoyed this puzzle, don’t forget to check out the list of the best math puzzles on Medium!
Math Puzzles
Explore a variety of math challenges, including Algebra, Geometry, Calculus, and Number Theory.
Feel free to share this with your friends and...
Thank you for reading! If you found this article helpful, please show your appreciation by clapping.
If you’re feeling generous, consider buying me a coffee. Your support helps me continue creating content for you. Happy Solving! 😊