An Infinite Landscape of Numbers

You’re likely familiar with the number line, a visual representation showcasing the locations of all numbers.

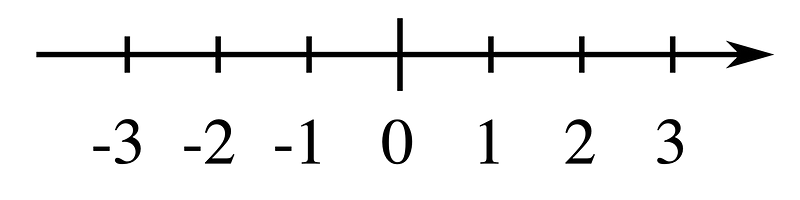
Each point along this line signifies a unique number. Imagine this number line as an endless map where you can easily find the address of any number that piques your interest.

Planning Your Journey

Once you've identified a number that intrigues you, how do you go about reaching it? Instead of conventional transportation, you'll need to use a different method—after all, this is mathematics!

For instance, let's say you're fascinated by the number 0.5. A clever friend has created a machine that can transport you to any number's location in no time. All you need to do is input a fraction, and off you go! But what should you enter? Let’s explore.

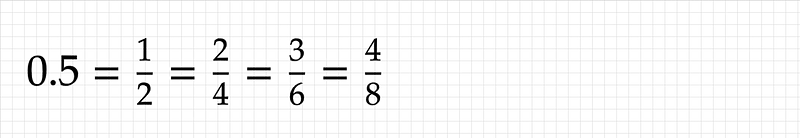
The example above illustrates four different ways to input the fraction for 0.5, but infinitely many options exist. Let's generalize how to specify these addresses.

Simply choose values for x and y, ensuring they are both integers. This results in a rational number. For our example, entering 1 for x and 2 for y will lead you directly to 0.5. You arrive, have a pleasant chat over coffee, and return home—a delightful outing!

The Challenge of Visiting Pi

After enjoying your first adventure, you decide to plan another—this time to meet the famous number Pi. After all, Pi is renowned, and you’d love to get an autograph.

So, you hop back into your machine and attempt to input Pi’s address. However, it seems you can’t reach Pi because it resides in a restricted area!

Despite Pi having a specific location, it cannot be expressed as a rational number. No matter which integers you choose for x and y, you can't pinpoint Pi's exact address. This is disappointing, but you're determined not to give up.

Getting Closer to Pi

Let’s examine Pi more closely.

Pi consists of an endless sequence of digits following the decimal point, with no discernible pattern. Numbers like Pi cannot be represented as a fraction of two integers, which makes them irrational.

So, what now? Are you left without options? Not entirely. While you can't directly visit Pi, you can certainly get close enough to observe. Think of Pi as a celebrity you can't meet, but you can snap some pictures from a distance.

Eager to see how close you can get, you input your first coordinates.

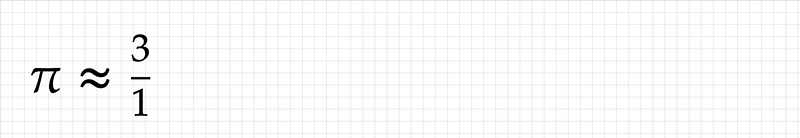
You enter 3 and 1, bringing you near the location of the number 3. You're not quite at Pi's doorstep, but you're close enough to take a peek.

It appears Pi resides on a lovely island, prompting you to try to get even closer.

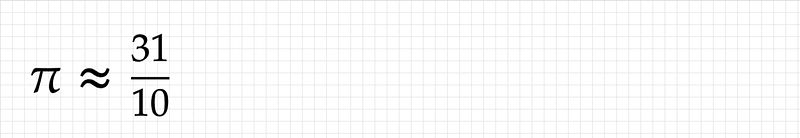
You enter 31 and 10, which brings you to the location of 3.1. Now you're much closer to Pi's island, confirmed by a quick binocular check.

Satisfied with your progress, you make one last attempt to edge even closer.

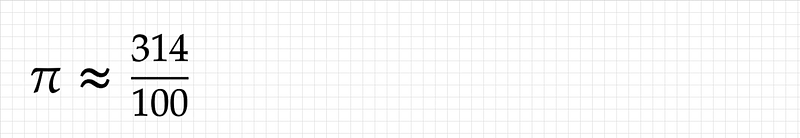
Now, you arrive at 3.14, getting closer to Pi than ever.

After soaking in the beautiful scenery, it’s time to head home. Although you didn’t get to shake hands with Pi, you captured some fantastic views.

Conclusion: Key Takeaways

What can you glean from this whimsical tale? Essentially, two main ideas:

1. Many points on the number line correspond to numbers that can be expressed as fractions of two integers, known as rational numbers.
2. Conversely, certain points represent numbers that cannot be expressed this way, referred to as irrational numbers.

To wrap things up, here’s a simple theorem to remember:

Irrational numbers are locations on the number line that cannot be accessed through integer fractions. They inhabit islands that are off-limits. While you can approach any irrational number by choosing larger integers for your fractions, you’ll never truly set foot on its island.